
This essentially boils down to finding the length of the curve (function), multiplying it by a constant and the function itself, and then integrating over the interval of interest to find the surface area.Īdditionally, by taking the coating's weight per unit area into account, we can then determine how much weight is added to our system when the coating is applied to the surface area of interest. If we are designing a bell shaped rocket nozzle that is parabolic in shape, we can take the function for the inner profile of the nozzle and revolve that profile around an axis to find the surface area of the nozzle's inner surface. Ablatives are commonly used on space vehicles and launch equipment to protect critical components under the resulting thermal loads seen during launch and flight. A substrate is the material that the coating is applied to.

What if we are designing a rocket nozzle and want to find out how much ablative coating is required for the inside of this nozzle?Īn ablative coating protects a substrate that is exposed to high velocity, high temperature gases and particles for a finite amount of time. We can use this concept for more than just completing some math problems. Step 1: Convert the centimeters into meters and degrees to radians.Why do we Learn About the Length of a Curve?įinding the length of a curve is another useful tool for our problem solving toolbox. What will be the arc length if the sector area is 360000 cm 2 and the angle is 60 degrees. Now, take the square root of the value.Ī solved example for this case is given below.When the available data does not include the radius then it is possible to find arc length using the sector area of the circle.
#Arc length calculator calculus how to
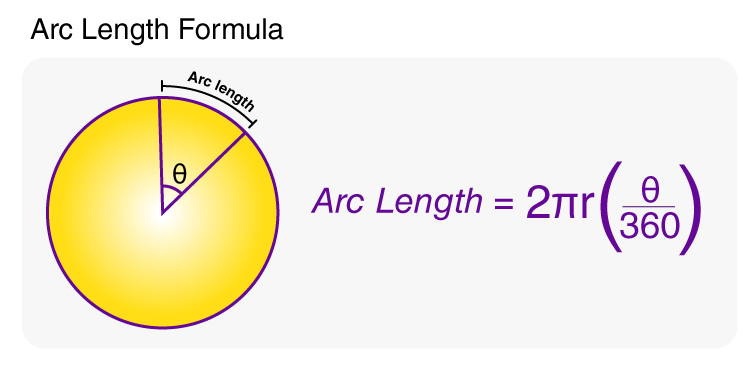
L = 9.774 meters How to find arc length using sector area and central angle? Since the angle is in degrees, we will use the degree arc length formula. After that, multiply both values.įor a circle of 8 meters, find the arc length with the central angle of 70 degrees. It is important to convert the units of the angle and radius in the SI unit. The arc length calculator can find the arc length in whichever unit you provide the angle e.g arcminutes or pi radians. But if the angle is measured in degrees, then multiply the angle with π/180. This formula is applicable when the central angle is given in radians. This is the simplified formula of arc length. Now, the circumference of a circle can be written as 2πr as well, right? So the proportion becomes: Where L is the arc length and theta is the central angle.
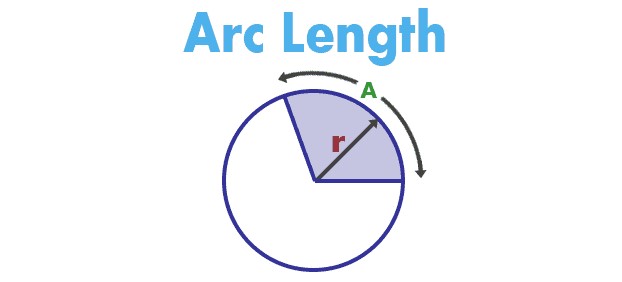
This means the proportion between these two values is constant. As you may already know, for a 360 (2π) degrees angle, arc length is equal to the circumference. Let’s derive the formula to find the length of an arc of any circle. Visit our other circle-related tool e.g Circumference and Circle area calculator. It is different from the chord of a circle because a chord is a straight line between two points while an arc is curved. It depends on the central angle and the radius of the circle. The length along the curve of a circle between two points of its circumference. This tool also allows choosing the units of the input quantities because they are of significant importance when computing some value. Arc length in radians calculator computes the arc length and other related quantities of a circle.


 0 kommentar(er)
0 kommentar(er)
